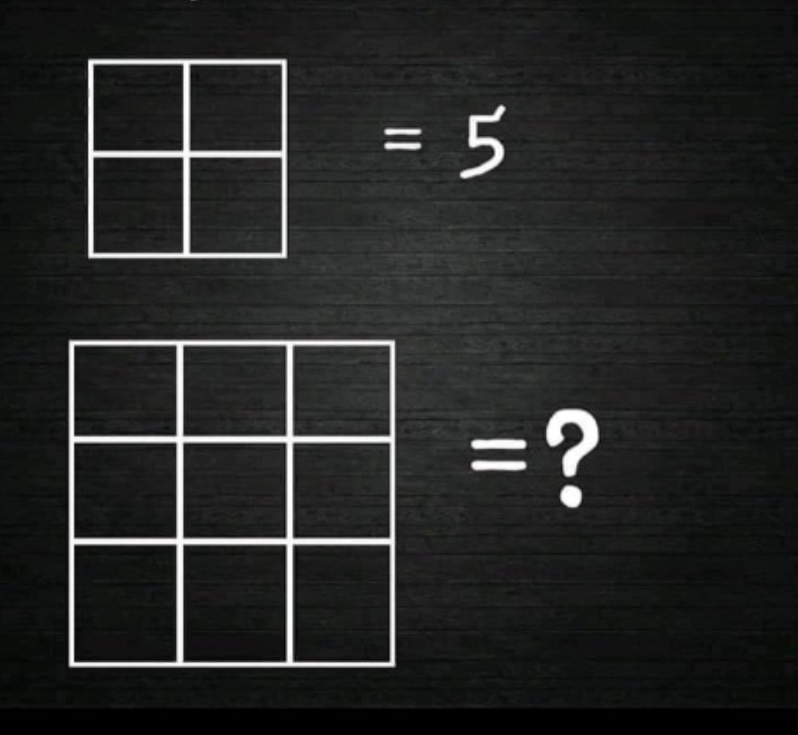
Are you ready for a challenge that will twist your brain and test your ability to spot patterns? Today’s puzzle involves a simple grid, but don’t let its simplicity fool you. This is a perfect example of how everyday puzzles can enhance critical thinking and problem-solving skills. Take a look at the image below, where a small grid equates to the number 5. The question is, what does the larger grid represent? I encourage you to pause here and give it a try before reading further.
When approaching puzzles like this, many people fall into similar traps. Here are a couple of common errors to watch out for:
- Miscounting Elements: Often, solvers count visible elements without considering the implied or hidden components of a structure. It’s crucial to consider every part of the puzzle’s makeup.
- Overcomplicating the Solution: There’s a tendency to think that a solution needs to be complex when, often, a straightforward approach works best. Sometimes, the answer lies in plain sight, and simpler arithmetic or counting methods are all that’s needed.
Now, let’s break down the puzzle step by step:
- Understanding the Basic Unit: The first image shows a 2×2 grid, and the equation states it equals 5. To understand why let’s look closer. The trick here is to see not just the four small squares but also the larger square formed by the grid itself.
- Counting All Possible Squares:
- Small Squares: There are four individual 1×1 squares within the grid.
- Large Square: The entire grid forms one larger square.
- Applying the Same Logic to the Larger Grid:
- Small Squares: In the 3×3 grid, you initially see nine small 1×1 squares.2×2 Squares: Count the number of 2×2 squares that can be formed. You can form these by grouping different combinations of four small squares, resulting in four 2×2 squares.Large Square: The entire grid itself forms one large 3×3 square.
Now that you’ve seen how to solve this puzzle, why not try to apply this method to other similar puzzles? Counting and pattern recognition are invaluable skills that can help not just in puzzles but in real-world applications like software development, architectural design, and more.
I encourage you to comment below with your answers or share other interesting puzzles that you’ve encountered. How did you approach them? What other techniques do you find effective? Let’s keep challenging our brains and sharpen our problem-solving skills together!
